Year 10 Trigonometry (Basic Introduction)

Year 10 trigonometry doesn’t need to be complex, although some teachers might make it feel that way.

Trigonometry comes from the Ancient Greek words trigōnon (triangle) and metron (measure), and it is exactly that: a way we can measure triangles, both the length of the sides and the angles between them. Even if it is not practical to physically measure all sides and angles we can use some unique properties of triangles to calculate the information we don’t know.

Understanding trigonometry can be incredibly useful in real life; whether it is measuring the height of building or slope of a roof, or in another application like navigation, engineering or criminology. Trigonometry has a large range of applications in different careers.

Right-Angled Triangles

Today we are looking at right angled triangles: that is a triangle when two sides meet at 90 degrees like the one below:

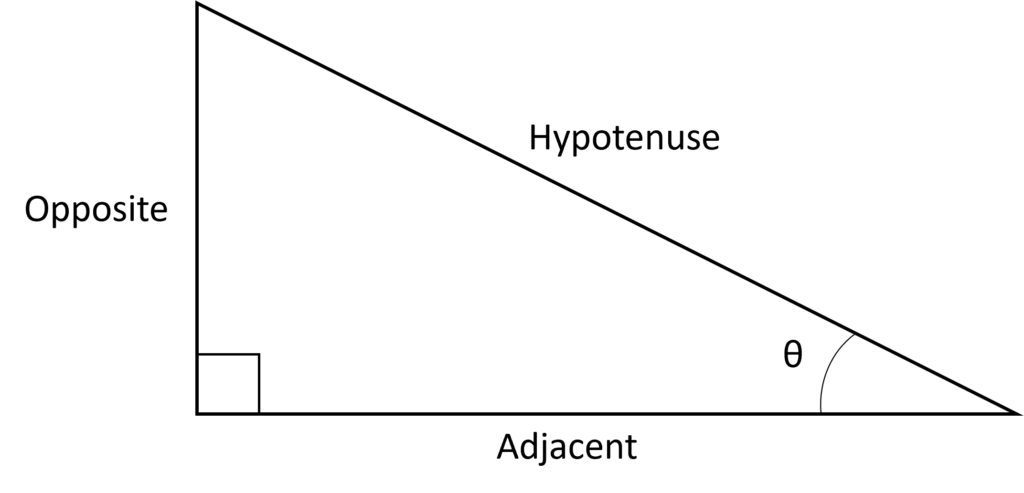
We call the longest side of a triangle the hypotenuse. When we either have or are looking for an angle in a triangle, we call the other two sides are called the opposite and the adjacent side. It is going to depend on context as to which is which.

For example, if we know or are looking for an angle (let’s label it θ), we call the side directly next to the angle the adjacent side, and the one opposite the angle the opposite side.

In the next section we will abbreviate hypotenuse to H, adjacent to A and Opposite to O to make it easy for us to remember.

SOH CAH TOA

When calculating information about triangles we can use sine, cosine and tangent to help us. They are written in equations as sin, cos, and tan.

We won’t be diving deeply into their meaning today. For a basic introduction it is sufficient to find them on your calculator and know when and how to use them.

SOH CAH TOA is a way of remembering which of these we should use when calculating our missing information about our right angled triangle.

SOH helps us remember sin(θ)=opposite/hypotenuse

CAH helps us remember cos(θ)=adjacent/hypotenuse

TOA helps us remember tan(θ)=opposite/adjacent

Which one we use will depend on what information we have and what information we are looking for.

For example if we know an angle and the hypotenuse and want to find out the length of the side opposite the angle, we can use sin(θ)=opposite/hypotenuse. Where as if we know the lengths of the opposite and adjacent sides and are looking of the angle we would use tan(θ)=opposite/adjacent.

Correctly identifying the information and writing out your problem is the hardest part. From here we are just solving a function much like you have done in the past.

Example: Finding the Length of a Side

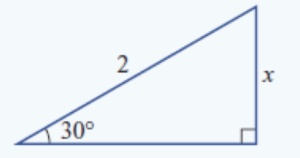
Maybe we know the angle is 30 degrees and the hypotenuse is 2 but we are looking for x.

x is opposite the angle so we would use sin(θ)=opposite/hypotenuse.

sin(30 °)=x/2

x= 2 x sin(30 °)

x= 2 x 0.5

x = 1

Another Example: Finding the Angle

Let’s say we know the length of the side opposite the angle, and the length of the hypotenuse but we are looking for the angle itself. We have O and H so we need to use sin(θ)=opposite/hypotenuse to calculate the angle.

Suppose the length of the opposite side is 4 and the length of the hypotenuse is 8. The value of sin(θ) would be as follows:

sin(θ)=4/8

sin(θ)=1/2

θ=sin⁻¹(1/2)

θ=30 °

A note that to inverse the sine function (sin^-1) you will most likely have to press shift on your calculator.

You may have noticed this is the same triangle as in the previous example.

Basic Take Away

When looking at a right-angle triangle, we can use SOH CAH TOA to help us find out information we are looking for.